Pathways to research: Young scientists tackle abstract problems
Students’ independent, theoretical investigations produce real-world results
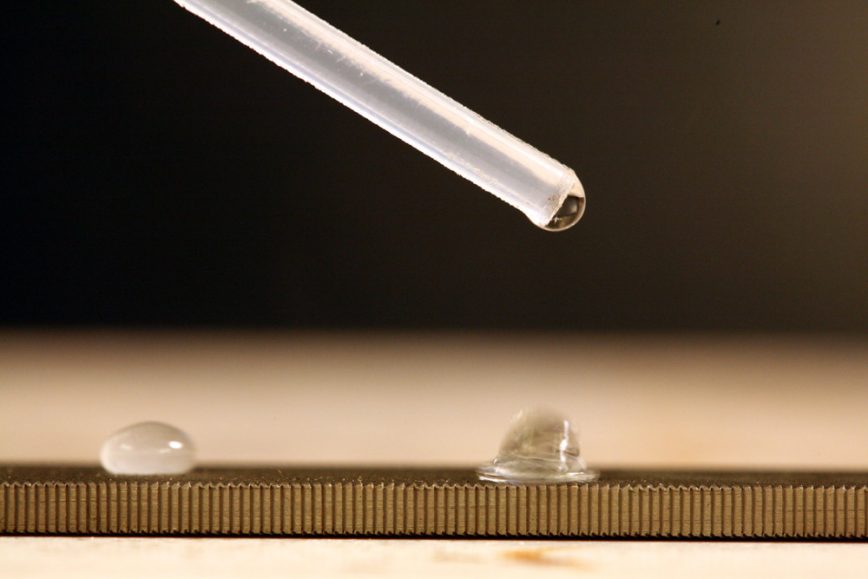
Dropped from a pipette, water droplets race across a heated hardware file.
Courtesy of Nilesh Tripuraneni
When Nilesh Tripuraneni set out to make pancakes one morning, he had no idea he’d also wind up with the makings of a first-rate science fair project. But as the high school student sprinkled water on a hot griddle to test its temperature, the dancing droplets got him thinking: What, exactly, was going on beneath them?
The sophomore at Clovis West High School in California turned to the Internet for clues to the hopping water droplets. He learned that when the surface of a griddle becomes very hot — much hotter than the boiling point of water — the lower part of water droplets can vaporize, or turn to gas, before reaching the griddle. That cushion of vapor allows the rest of the droplet to dance.
Tripuraneni also learned about researchers at the University of Oregon in Eugene who had discovered a way to make the droplets race across a heated, grooved surface. This movement really fascinated Tripuraneni, who has always loved fluid mechanics. That’s a branch of physics that deals with liquids and gases. Before long, the teen was at work describing the racing water droplets mathematically.
Building on such inner passion is the best way to start a research project, according to Dave Marker, chairman of a judging panel for the Intel Science Talent Search. The Intel STS is a research competition sponsored by Society for Science & the Public, which publishes Science News for Kids.
“I’m impressed by theoretical projects where students have been fascinated by the area — reading and working around it long before they begin the project,” says Marker.
Theoretical projects like Tripuraneni’s are abstract; here, that means they use mathematics to understand the relationships between objects. Such studies use equations and, in some cases, computers to simulate, or model, how objects move in space and time under various conditions. Most of these projects investigate the physical world using known properties of chemistry (how molecules react with one another) and physics (how energy and matter interact).
One advantage of theoretical research: It usually does not cost a lot of money because it requires little equipment other than a family computer. Another perk, for some: Theoretical investigators can work largely alone.
Here we focus on the research pursuits of Tripuraneni and other young scientists who have chosen to do theoretical research.
File away
Tripuraneni began his investigations by re-creating the Oregon team’s experiment. He didn’t have access to the equipment needed to cut grooves into metal, the way the Oregon researchers had done. But he soon found a promising substitute: a carpenter’s file. This tool is a long piece of metal with crosshatched or other deep grooves on its surface. It’s normally used to shave rough surfaces off of a piece of wood or metal. When Tripuraneni heated the file, water droplets raced along its grooved surface, just as they had done for the Oregon team.
Before he could fully understand and describe that movement, he needed to know more about the shape of the droplet and the thickness of the vapor cushion that developed on top of the heated file. To do this, Tripuraneni took photos of the droplets and measured them using a computer programming language called MATLAB. But first he had to teach himself MATLAB — a long process of trial and error.
During his initial experiments, Tripuraneni noticed that the bottom edge of the droplets and the surface of the file acted like two parallel plates. That gave him a good starting point for his calculations, since there already existed a model — or simple set of mathematical equations — describing that condition. Tripuraneni then developed his own model to describe the movement of the vapor slipping between those two surfaces.
Finally, he plugged the MATLAB measurements into his new mathematical model to test it. By tweaking his model, Tripuraneni found that it eventually described the movement of the droplets across the surface of the file. His work nabbed him second place in the 2007 Intel International Science and Engineering Fair (also sponsored by Society for Science & the Public). Today, the 21-year-old scientist is a student at Harvard University.
Drawing a mental picture
Most people find it hard to describe a physical surface or interactions using math — until they see it or somehow picture it. It certainly helped John Pardon when he was a high school student at Durham Academy in North Carolina.
He loved math, so he spent much of his spare time reading math papers online. At some point, he discovered a list of unsolved problems in differential geometry — a branch of mathematics that deals with curves and surfaces. One problem on the list really got him thinking: It asked whether it was possible to take any smooth curve in a closed shape and round it outward — or make its shape convex and closer to that of a circle — without stretching the curve.

To make it easier to investigate the idea, Pardon pictured a loop of string on a flat surface. The edges of the string curled in on themselves, but did not cross or touch. Then he imagined how the string could change shape.
Studying this mental image, Pardon became sure there had to be a way to round any smooth curve. A group of mathematicians — Robert Connely, Erik D. Demaine and Günter Rote — had recently solved a similar problem for polygons, which are closed shapes bounded by straight lines. But no one had solved the same problem for curved shapes. With the solution to the polygon problem in hand, Pardon decided to take a similar approach to his curve problem.
Pardon worked through a number of approaches. None worked. Eventually, he decided to seek help. He turned to Robert Bryant at nearby Duke University, a specialist in differential geometry.
Experts often help people new to some field of study by serving as a mentor — sort of a coach or a person who helps guide another, without actually instructing them about what to do. Mentors often play an important role in research projects, even those that are tackled by someone working alone. “It’s very difficult … to get started on a high-level theoretical project without a good mentor,” says Marker. Mentors’ occasional advice, he notes, can ensure that no basic points are missed.
When Pardon met with Bryant, he found an expert with a willing ear. In explaining to Bryant what he was doing, the teen figured out which parts weren’t working — and which parts he needed to think over more carefully. Bryant didn’t tell Pardon what to do. Rather, Bryant just guided the teen’s understanding of the problem by asking helpful questions. Afterward, Pardon headed home to try something new. Again, things didn’t work. So he found himself repeatedly meeting with his mentor to explain his frustrations and failures.

Whenever Pardon got stuck, he re-read the paper that solved the problem for polygons. It was easy to read and often gave Pardon ideas for how to alter his own work. Step by step, he got closer to a solution.
The hard work finally paid off: Pardon solved the problem. His accomplishment earned him second place in the 2007 Intel STS and his work was later published by the American Mathematical Society. Today, at 23, Pardon is working toward a PhD in mathematics at Stanford University.
Trial and error
To Clara Fannjiang, 18, “the definition of doing [independent] work is trial and error.” The daughter of a math professor, she has always loved applied math — that used in science, technology or other areas. During her second year at Davis Senior High School in California, she took a college-level class in linear algebra; this is a branch of math that deals with vectors and their locations in space. She quickly realized that this form of math could be used to process digital data. Those are data recorded as a series of zeros and ones — the type that can be easily used in computing.
And the young woman knew just what signals she wanted to study: radio emissions from faraway galaxies.
Throughout high school, Fannjiang often read up on astronomical research for fun. She discovered that the Very Large Array — a set of enormous radio telescopes in New Mexico — required huge amounts of digital data to form images of galaxies. Each of the Very Large Array’s 27 radio telescopes is a massive dish antenna. Picture a monster-sized version of the satellite dish you might have outside your home — but tuned to receive radio signals from distant space.
Unlike most large radio telescopes, those in the Very Large Array can be moved along a set of tracks. When the telescopes are close together, less than 1 kilometer (3,280 feet) separates them. When they are spread out, the farthest antennas are 36 kilometers apart. Astronomers combine data from all of the telescopes to obtain detailed images from space. But combining those data points to get even one image can take hours. So Fannjiang looked to develop a better method of collecting and processing those data.
She didn’t have access to the Very Large Array, so her first step was to use MATLAB to create a mathematical representation of how the telescopes collect data. “That was my biggest hurdle,” she explains, because she had no experience with computer programming. She spent six to eight months figuring out how to use the linear algebra she had learned to create a computer-generated version of the Very Large Array’s measurement process. Once she had a working simulation, or “model”, she applied them to galaxies.
It wasn’t long before she discovered that she needed other types of math as well. Fannjiang turned to her dad, who recommended some good textbooks. As she got up to speed in each one, she was better able to work with the computer-simulated galaxies. By tweaking the mathematical equations used in her computer code, the teen was able to play with different telescope arrangements and evaluate the quality of the image that would be produced by each.
After 18 months of work, Fannjiang identified the best way to arrange the Very Large Array’s radio antennas to reduce data processing time and to provide clearer images. She believes her findings will help radio astronomers. Her work was published by the Society of Exploration Geophysicists, and she was named a finalist in the 2012 Intel STS. Fannjiang currently attends Stanford University.
Igniting the spark
Sticking with a project long enough to wade through the ups and downs — temporary successes and failures — requires finding a topic that ignites a spark of excitement. “Do what your brain and your mindset are wired into,” says Fannjiang. In other words, don’t limit yourself to some project simply because it’s convenient or easy. Do what truly excites you.
Tripuraneni agrees. “The key to a great experience is picking a good project — interesting, very doable and well-suited to the student,” he says. Making a theoretical project doable often means starting out with simple mathematical equations, he explains. The solution doesn’t have to be complex. In fact, simpler solutions often work better than more complicated ones.
Pardon, Tripuraneni and Fannjiang all agree that reading widely and taking courses, if necessary, in subjects not available in your school may be key to tackling the problems that most interest you. And if needed courses aren’t available, there are other options. Tripuraneni and Fannjiang taught themselves much of what they needed to know (including how to program a computer using a specialized computer language). And when Pardon ran out of math classes at his high school, he set up a one-on-one course with his math teacher.
Talking with experts can also be a huge help, notes Andrew Ferrari, the teacher who taught Pardon. Not only do these experts know the latest developments in a particular field of science, but they also tend to have ideas for a wide range of research projects that an interested student can take on.
Although all three students spent a lot of time actively working with mathematics, they also did a lot of sitting and thinking. Fannjiang spent about half of her research time debugging the code in her computer programs. At times, she spent literally hours searching for that one problematic segment before correcting it and then re-running the simulation — only to find new glitches that needed to be fixed. Frustrating as that is, “you have to work through it,” she says. “Don’t be afraid to do things you could be a total idiot doing the first time around.” Eventually it really does get easier, she says.
The long hours paid off, and not only in terms of science fair recognition.
“I’d say the best part of doing the project was being able to apply — and really think hard about — the techniques I had learned the previous year in the [one-on-one] advanced class,” says Pardon.
Tripuraneni loved having the chance to learn a lot about so many different things. “Not all of my research projects have been successful, but I usually end up learning quite a bit along the way,” he says.
And Fannjiang was thrilled to step in and work with a real-world application. She’s not planning a career so she can later do this kind of research — she’s already doing it today.